Danielle, a 6th grade teacher at my school, recently shared this March, 2018 blog post from Robert Kaplinsky. I feel his point and the embedded TED Talk from Conrad Wolfram are just as relevant 5 years later. Unfortunately, very little in math education has changed since 2018 in regards to the amount of hand calculations we still are required to teach and assess.
NCTM Los Angeles, 2022
Key Takeaways From NCTM Los Angeles 2022
by Jeff Linder
- Where are all the teachers? The last NCTM had 12,000 attendees. This year there are only 2,000. This is at a time when there is substantial monetary support for professional development. Is it due to the shortage of substitute teachers?
- Peter Liljedahl says:
- that thinking is a precursor to learning.
- diversity is a strength in group thinking.
- students listen to teachers’ actions, not their words.
- one way to show students that we believe in them is to put them in random groups instead of teacher-selected groups.
- Jay Meadows from Exemplars reminds us to build curiosity in the math class.
- John Felling introduced me to many fun math games that promote both thinking, strategy, and skill practice. My favorite was Who’s In Between.
- Graham Fletcher highlighted the importance of a balanced math program with application, conceptual understanding, and procedural fluency. He presented a 3-Act task for the application, followed by a tool talk for the conceptual understanding, and ended with a task from Open Middle for procedural fluency.
- Jennifer Lempp presented on the Math Workshop model. Her presentation confirmed that all the principles of math workshop are alive in MUS classrooms.
- Christine Franklin from the American Statistical Association shared some great resources for teaching statistical concepts, such as mean and variability.
- Jo Boaler presented on the new California Math Framework.
- The Framework was scheduled to get approved in 2021 but due to pushback it will not be approved until at least 2023.
- The three main areas are big ideas, all students having access, and data literacy.
- Currently, only 16% of students are taking Calculus in California high schools. This is an example of institutional racism that the framework is working to change.
- Traditionally, passing Calculus in high school has been a prerequisite to attend top universities. Harvard no longer require calculus for admission. “Specifically, calculus is neither a requirement nor a preference for admission to Harvard.”
- Data science is now an accepted replacement algebra 2 courses.
- Groupitizing 10 dots, for example into 4+4+2, is a predictor of math achievement. My takeaway is to do more dot card number talks at all grade levels!
- I am grateful for the opportunity to learn from so many amazing educators. NCTM puts on a great conference, maybe next year more teachers will take advantage of it.
CMC South 2021 Reflections
Image
CMC 2021 was AMAZING! Is was so great to attend an in person conference with so many great presenters. Here are some of my biggest takeaways:
- Attending a conference with a team of thinking partners makes it so much more valuable. Thank you Abbey Shaw Linder and Kristy Guerrero for spending two amazing days with me.
- The California Math Framework vote for final approval was delayed until July 2022. That will have an impact on the State math textbook review and adoption timeline as one of the functions of the framework is to give direction to textbook publishers.
- The textbook industry has taken a strange twist. The Gates Foundation funded the writing of a free and open source program in math, as well as other subjects. Our 6th grade has been using the free Open Up program for several years. Now several textbook companies have made a few minor changes and have repackaged that free program and have put it up for sale. Kendall Hunt, Desmos, McGraw Hill, and Amplify have or are in the process of repackaging that free program and selling it on the open market. I wonder how many districts will adopt some version of Open Up?
- Jen Hunt’s All Gain Access Through Play: 8 Games for Learning Recovery workshop reminded that some of the best intervention and practice is in the form of games but sometimes the competition can cause kids to shut down. Turn competitive games into cooperative games to get the most from students in this setting.
- For students who have not mastered a skill, some just need more time and practice while others need more prerequisite knowledge. For student who need prerequisite knowledge go back to concrete and conceptual understanding.
- Steve Wyborney says, “the person doing the talking is doing the learning.”
- Cathay Williams says do creative mathematics together to build a community of learners. She also emphasizes making learning visual, like this:
- Robert Kaplinsky used 162 slides to teach me that the best problems and tasks use many of these elements: simple, unexpected, concrete, credible, emotional, and stories.
- Annie Fetter reminded me that answers are one of the least interesting part of math class. How we get those answers is among the most exciting part of class.
- Graham Fletcher created some really great math progression videos https://gfletchy.com/progression-videos/
- Peter Liljedahl’s Building Thinking Classrooms in Mathematics is a MUST READ. The book describes 14 teaching practices to enrich math learning. He spent 15 years observing over 400 teachers and experimented in two week cycles to come up with this list:
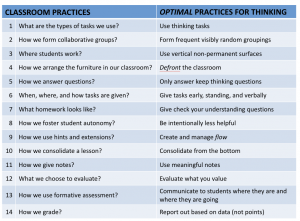 . In number 1, a thinking task is as simple as using a problem for your textbook, before you teach the lesson.
. In number 1, a thinking task is as simple as using a problem for your textbook, before you teach the lesson. - Annie Fetter taught me that when kids are stuck, have them go back and do more noticing and wondering.
- Annie Fetter also suggested that we build in notice and wonder after each number talk to allow students time to process the strategies and student thinking displayed on the whiteboard.
- CMC puts on an amazing conference. The top notch presenters, exhibitors, and attendees have made a lasting impact on my teaching.
Lesson Study
In November 2019, I attend my 3rd CANMEE lesson study training. “Lesson Study is a Japanese model of teacher-led research in which a triad of teachers work together to target an identified area for development in their students’ learning. Using existing evidence, participants collaboratively research, plan, teach and observe a series of lessons, using ongoing discussion, reflection and expert input to track and refine their interventions.” (Teacher Development Trust) In short, lesson study is a professional development model where teachers plan, teach, and reflect together around an identified goal or focus. The key piece is one or more shared classroom experiences teaching real kids.
In the United States, the typical professional development happens in a school cafeteria, office building, often associated with a county office of education, or in a hotel ballroom or convention center in the form of a conference. These professional developments are led by teachers or more often by former teachers that have been removed from the classroom for a number of years. I am one of those teachers that has not lived the life of an everyday teacher for 9 years. The critical piece that is missing from this professional development model is the students. I can sound convincing talking about the latest research and teaching strategies with colorful slides directed at the teachers. But does it really work with students?
Lesson study incorporates teacher learning and training with real students. Students who are not robots and do not always respond or behave in predictable ways. In November, I attended the lesson study conference with Fawn Nguyen, Melissa Wantz, and Margarita Mosqueda. Three AMAZING classroom teachers that are now in coaching roles. After spending 2 days learning about lesson study, we decided to give it a try. We reached out to two third grade teachers, Erika Padilla and Jaqueline Leal. We targeted Erika and Jaqueline because they were two teachers that we have a good relationship with and are open to trying out new ideas. The two teachers were willing to give up a few hours or their after school time to let us experiment with lesson study.
For Fawn, Melissa, Margarita, and myself, we wanted to know what was the big deal about lesson study? Is it doable in our current structure? Is it a better model for professional development?
We met in December to determine our learning goal, when the public lesson would take place, and logistics we needed to work through to pull this off. Last week, the team of 6 met to plan a 3rd grade lesson on elapsed time. We started with the districts adopted math curriculum and picked the lesson that fell on the day of the public lesson. We call it a public lesson because it a lesson that we all observe together, with one of us teaching. In addition, we could invite other teachers, parents, and administrators. With this being our first attempt, we stuck with the six of us.
Besides students meeting the publisher and Common Core learning goal of calculating elapsed time, our team wanted to focus in on students communicating their thinking. We read through and dissected the publisher lesson. It was a strong lesson but we wanted to ensure we connected more to students. We felt that the more the students could talk about elapsed time in their own lives, the more they would connect to the lesson and the more engaged they would be. With the learning goals in mind, the six of us modified the lesson to meet the needs of the students.
Today was the public lesson. We went into the process hoping Erika or Jacqueline would volunteer to teach it. Understandably, they deferred to me. It was a new process with a group of highly respected peers observing. The safe thing was to let someone else teach. The exciting part for me was that I got to teach the class that has by twin 9-year old boys. It was a fun crossover between my professional and family life.
Immediately following the lesson, the six of us met in an empty room to debrief. Besides getting to see my two boys as math learners, the reflection process was the highlight of my morning. Fawn played the role of the content expert. She focused her observations on the mathematics and the students’ strategies. Margarita focused on how our two focus emerging bilingual students responded to the instruction. Melissa, whose expertise is in Language Arts, provided an outside perspective and led as the facilitator. Erika and Jacqueline provided the perspective of the teachers that work with these kids and teach the mathematics curriculum on a daily basis. Their feedback and reflection was a gift, one of the most influential professional learning experiences I have had in years. I feel all six of us grew significantly as educators today.
We ended our time by reflecting on the lesson study process as a whole. We all agreed it provided an amazing professional growth opportunity because of the planning and reflecting around a common experience with real children. Some of our other takeaways:
It is so valuable to take your time early in the year to set up your classroom norms, routines, and learning community. I benefited today as the classroom teacher because of the work Erika put in to establish those norms, routines, and learning community.
Knowing and connecting with your students matters. Being an outside guest teacher left me without the student connection, without knowing the individual student’s strengths and needs, and without them having that connection with me.
Although we want right answers, wrong answers provide more opportunities for rich mathematical discussion and student learning.
Provide opportunities for students to share their thinking with each other and take the time to truly listen to their thinking without judgement.
Let the students own the math and the learning.
We asked Jacqueline and Erika about their perspective about lesson study as a model for professional development. They asked for more and offered to take on the role of the teacher next time. Margarita, Fawn, and Melissa will continue to explore lesson study as they are all content specialists in the same district.
I want to wrap up this post with a HUGE thank you to Erika, Jacqueline, Melissa, Margarita, and Fawn for the opportunity I had to learn from them and to grow as an educator. Their willingness to share their diverse experiences and expertise helped me grow as a classroom teacher and as a person that supports classroom teachers in the area of mathematics. There are so many reasons we can generate for why the Japanese lesson study model won’t work in the US. Everyone of those reasons is worth overcoming so that teachers can learn with their peers, in front of real students. Afterall, the students are why we are here.
Is My Child on the Right Pathway to College Readiness?
One of the many decisions parents and families grapple with as their children reach 5th and 6th grade at MUS is where to send their children next? In our community, that includes a variety of public and private school options. For years, we have been able to create a system that allowed students to seamlessly flow into either the public or private school setting. In 2013, the shift to the Common Core State Standards made that more challenging, specifically in the area of mathematics.
The Common Core State Standards are essentially a checklist of skills and behaviors that students need to be able to do or demonstrate at each grade level. The change from the previous California State Standards to the Common Core State Standards was a revision of that checklist that guides teachers and curriculum makers. In K-7th grades, those changes were mostly minor revisions. Maybe a skill or two moved down a grade level, but the list of skills stayed the same. A major shift was the added expectation that mathematics was to make sense. Memorizing the steps to solve a problem is no longer sufficient. With the addition of the Standards of Mathematical Practice, students are expected to understand why the math works and when to apply it.
An additional shift that has had a significant impact on 7th-11th grade is the choice to teach math in the traditional pathway where each domain (algebra I, geometry, algebra II, and precalculus) or in an integrated pathway where algebra, geometry, and statistics are taught in each grade level 6th-11th grade. Essentially the standards from the traditional pathway are shuffled together to teach a fraction of each of the individual domains each year, in an integrated way. Rarely does an individual domain show up in the real world in isolation. By teaching in an integrated way, teachers have the opportunity to provide more sense making opportunities for their students and more opportunities to apply mathematics to the outside world.
The decision to continue with the traditional pathway or shift to the integrated pathway was left up to each individual district in California. The majority of the districts in California, under the recommendation of the California Math Framework and many leading math educators, shifted to the integrated pathway. This is also the methodology chosen in most other parts of the world. In fact, the American “traditional” system is not used in many other places as it makes these connected “maths” seem like separate subjects rather than parts of a whole. At this point, the majority of the private schools have maintained the traditional pathway, which has made it much more difficult for schools to align with both the public and private schools at the same time.
As mentioned above, the changes in K-7 were relatively minor. So, why would this affect the transition to 7th grade in private or public school? For most of our students, it does not. Most students seamlessly transition to either the integrated pathway in the public schools or the traditional pathway in the private school. Our students excel in both.
The shift does have an impact on our extremely advanced students compared to their peers who graduated MUS before the introduction of Common Core. This small group represents students that score near perfect on state testing and are our Math Superbowl finalists. Before Common Core, these students would entirely skip 5th-grade content standards. In 5th grade, they were grouped together all year and taught the 6th-grade curriculum. In 6th grade at MUS, they were taught the 7th-grade curriculum. These students left MUS and fed into 8th-grade Algebra I as 7th graders in public or private school. Around 2015, with the adoption of the Common Core State Standards, the local public school that our students feed into, developed the integrated pathway, where all income 7th graders enter into 7th-grade math. In that pathway, students choose to enter into 7th-grade math, 7th-grade honors, or 7th-grade compaction were 3 years of content standards are taught in 2 years, instead of 3.
Besides the change in our local feeder district, as stated earlier, the Common Core State Standards added a layer of complexity with the Standards of Mathematical Practice. Students are now expected to understand the math at a deeper level as opposed to just memorizing steps and shortcuts to compute. We have decades of international research to prove that the traditional approach to teaching left Americans behind most of the world in the area of mathematics. Just think, if you were given an Algebra II final exam today, what score do you think you would get? That score is not a reflection of your abilities but rather a byproduct of how we learned math. Providing opportunities for critical thinking and real-world application has required us to slow down. Entirely skipping 5th-grade math is no longer an option as we would undermine the critical foundation that our students need to succeed in advanced mathematics. That is the major issue still challenging the 7th-grade math compaction teachers in the middle schools.
Also around 2015, many new research studies were presented about the negative consequences of leveling students by ability. In those tracking systems, all subgroups underperformed compared to similar subgroups taught in mixed ability classes. With study after study showing similar results, and with the endorsement of the California Mathematics Project, National Council of Teachers of Mathematics, and leading researchers at Berkley, UCLA, Stanford, and Harvard, it forced us to evaluate our longstanding practices of how we grouped kids in mathematics at MUS.
Influenced heavily by the new pathways from our local public schools, new expectations set by the Common Core State Standards, and by new research on grouping students in mathematics, we changed our program at MUS. Starting in 4th grade, we stopped leveling our students entirely in mathematics. Instead, we created opportunities for differentiation within the classroom around depth instead of acceleration. After two years of positive state testing data, we slowly graduated out the leveled math classes and acceleration from our math program. We now teach math in all grade levels (K-6) in mixed ability classes. This shift has increased the workload for teachers as they now have to plan each lesson in new ways, thinking about different ability levels.
In 2013, the UC and Cal State schools issued a joint statement called the Statement on Competencies in Mathematics Expected of Entering College Students. They have found that the majority of students entering mathematics related studies were strong at computation and completing formulas but lacked the depth and understanding required to excel in mathematics at the university level. Many of those students skipped math their senior year because they competed Calculus AB as a junior. Many have since changed their admission expectations to encourage depth, understanding, and high performance over acceleration.
I often get asked from parents, what math class should my child take once they leave MUS. My only advice that I give is to backwards map so that their child has the opportunity to take Calculus AB as a senior. This advice keeps all doors open for students to be able to earn a spot in math-related majors at our top universities. The short term bonus is that students have time to see the beauty of mathematics and the real world application of mathematics. They get to love and appreciate mathematics because of that, not just because they are good at it. Long term, more of those students will want to major in math-related areas, will excel in their university courses, and go on to apply their skills to impact the world after graduation. Sometimes when you rush to get to the end as quickly as you can, you miss out on why you were on the journey in the first place.
How do we teach data literacy through the context of science inquiry?
In December, Dan Meyer asked during his keynote address at CMC North, “What’s Your Question?” He went on to highlight how math, science, and literacy all converge in the practice of critiquing reasoning. Given the rise of fake news and alternative facts we have a responsibility to educate children to be critical consumers of information and data.
Dan Meyer’s talk, along with insights from “playing” with the integration of science, math, literacy, and technology with my amazing colleagues Jennifer Wilson and Vanessa Scarlett, has lead me to my driving question, How do we teach data literacy through the context of science inquiry? From that, additional questions have surfaced. Can we completely remove data standards out of the math program and completely teach them in the context of science? Are there technology standards that support the work we are trying to do with data in science? Do the expectations of data literacy in NGSS and Common Core Math align? Do teachers have the content knowledge to effectively teach data literacy?
As we go down this road over the next several years, here are some of my initial thoughts and findings:
· The data standards in the mathematics standards are designed to be taught and developed in the mathematics classroom. The math standards at any specific grade level are not sufficient to get to the depth of science understanding and thinking that we are looking for. For example, bivariate data is placed in 8th grade so that students have the ability to calculate the equation of a line, slope, and have experience with coordinate grids. Students can and need to use a scatter plot to see patterns in bivariate data as early as third grade. Although calculating the line of best fit, slope, or equation for the line adds value and precision to the conversation, it does not warrant waiting until 8th grade. Our first hand experience with students validates this.
· The data standards are typically taught in the context of a math book and not involving real experiments and student generated data. We have observed what we have intrinsically known, data taught within context is much more accessible to all learners. “Because raw data as such have little meaning, a major practice of scientists is to organize and interpret data through tabulating, graphing, or statistical analysis. Such analysis can bring out the meaning of data—and their relevance—so that they may be used as evidence.” NGSS
· Some standards such as mean are not introduced until 6th grade due to the need for students to be able to divide using decimals. By utilizing technology (Google Sheets or Excel) students in 3rd grade can easily calculate the mean and have an understanding of what is being done, without having the mathematical skills to hand calculate it.
Keep in mind that we do not believe in teaching standards early because a student is high. We have spent a significant amount of time educating our teachers and parents about our philosophy of going deeper, not ahead. We believe the shifting of some of the data standards allows us to go deeper in our science understanding and depth of thinking and that these shifts do not undermine this philosophy.
Here is our first draft at creating a scope and sequence to teach data literacy in the context of hands-on science inquiry. We would love your feedback and suggestions.
8 Deadly Sins of Teaching Math
My “friend” Fawn Nguyen just posted
7 Deadly Sins of Teaching [Maths]
http://fawnnguyen.com/7-deadly-sins-of-teaching-maths/
I feel they are worth sharing. The first 7 sins come directly from Fawn. I added an 8th, because 8 > 7.
Giving extra credit. I don’t care where you teach, how old your students are, what your zodiac sign is, you’re going to have at least one kid who’ll ask for extra-credit “work” at the eleventh hour of the grading period. Don’t do it. Say no and walk away because the tears might come streaming down his/her face and you have to ration the use of Kleenex. And you should be ashamed of yourself for giving students extra-credit points for bringing in copy papers, sticky notes, dry-erase markers, tissue boxes, doughnuts. Yes, you should send me some.
Giving timed multiplication drills. Maybe there’s a well-documented success story behind this madness that I’m not aware of, but to me, it perpetuates the myth of faster-is-smarter. This practice raises self-doubt and affirms the why-should-I-even-bother mindset.
Giving out the equation. That’s like giving away life’s secrets to someone who flies to Paris to have lunch. Meaning, they don’t need it, nor did they ask for it. Your students’ conversations, their conjectures, their models — are all at the heart of a math class. To give away the equation is to passively (and aggressively!) dismiss our students’ abilities to think for themselves. It’s okay to eventually give them the equation in due time, just don’t start with the equation. Imagine if I just gave my students the equations for slope and area of a circle.
Teaching from one source. No one source is that good. The creators of that source would be fools to not concede that point. It’s like eating out at the same restaurant or boasting that you can make chicken 50 different ways. No you can’t, and nobody cares. Let one or two sources be your structural outline, your mainstay, then supplement it with your favorite lessons or other teachers’ favorite lessons. Remember, any well-crafted lesson outside of the textbook that you can bring in is your gift to your students. Tell them that. And with our prolific #MTBoS, you cannot afford not to supplement.
Talking, talking, you’re still talking. I pretty much end every workshop with this reminder: The more you talk, the less your kids learn. I plan each lesson using this as my go-to guiding principle. Math is a highly social endeavor, so for the love of Ramanujan and Lovelace, please stop talking so much so your kids may talk! Every question you pose is an opportunity for your kiddos to ponder [quietly by oneself first] and share their thoughts with peers. Every question! If you fret that your kids don’t talk in class, then I wonder about two things, 1) Do students feel safe enough to talk in your class? and 2) Is the question you’re asking interesting/worthwhile/challenging to even bother? (I must have asked hundreds of lame, boring, worthless questions, but I’m not giving up. I practice and get better.)
Keeping up with the Joneses. That colleague whose hair and complexion are always perfect is just not as funny as you are. That teacher whose students all adore her probably owns a cat that wants to kill her. And that “amazing” teacher whom everyone talks about probably sucks at everything else in life! And he might be a compulsive hoarder of all things creepy! So, don’t mind them. We’re not here to compete with one another. We’re here to make mathematics rock for our kids. There is one you and 24 hours in a day. Make time for yourself, make time for your family. We all have s#@$&y days that rob us of our wits and sensibilities, but recognizing that and committing to having a better day tomorrow are worthy endeavors. Our students need us more than they care to admit.
Being an a%$hole. No one wants to learn from someone who’s mean and angry and bossy. When we try to establish authority in the classroom, we may inadvertently end up being perceived as this person. The meaner we get, the less students want to have anything to do with us, so the angrier we get. It’s a vicious cycle, and everyone is losing. We’re the adult in the room, charged with a magnificent duty to establish a learning culture, which will not happen if we don’t behave like an adult. Children are said to be resilient, but they are also impressionable, and their impressionable minds are vulnerable — vulnerable to criticism, to shame, to false praises.
Giving mixed messages. Live the expectations you have for your students. Everything you do sends a message to your students. Send the message that you love math. Show them how to be curious, how to make mistakes, and how to learn from your mistakes and from the mistakes of others. Show them your wondering by asking “what if” about a problem the class has solved but you find interesting enough to take it further. Show them how to listen to the thinking of their classmates and how to ask questions to help you better understand their thinking. Show them how to be excited when presented with a challenging problem that initially looks way to hard to solve.
Technology Integration
In February, I facilitated a professional conversation with the UCSB Math Project Leadership cohort around the use of technology in the math classroom. My belief at the time was that technology was not valuable unless it allows me as a teacher, to do something better than I can already do without technology or provide access that was not previously available. With the help of the amazing workshops and presenters at NCSM, and presentations by Janet Hollister and Fawn Nguyen at UCSB my thinking has greatly changed.
Our students live in a digital world. They must be able to read, write, and think in that world. Our math classrooms must include blended learning opportunities. In my classroom, students engage in face-to-face problem solving with rich tasks, using real hands-on manipulatives, charting their thinking on paper, and presenting their thinking to classmates for constructive feedback. This is the norm. Although a few apps appear in my classroom, I do not teach in a blended classroom. I am not integrating technology; technology is just a guest visitor. Students need opportunities to read problems delivered on the computer, use virtual manipulatives, record their thinking on the computer, collaborate with their peers and receive feedback on the computer, and use technology to do the calculations.
My thinking has changed and my classroom needs to change. Doing these things digitally did not meet my previous belief about technology integration as I was already doing these things as well, using my old school environment. I now believe that students need to be able to operate in both the physical, face-to-face world and in the digital environments even if it is not “better”. Higher education, the job market, and our students demand it. I have changes to make and learning to do!
My next post – If you’re not doing a rich task, the platform (paper and/or digital) is not the issue.
NCSM Highlights
I just finished 3 full days of NCSM (National Council of Supervisors of Mathematics) Conference in Oakland. There were so many amazing presenters that I did not attend presentations by Jo Boaler, Dan Meyer, Matt Larson, Bill McCullum, Cathy Seeley, Deborah Ball,…
Here are highlights of some of the presentations I did attend:
• Jason Zimba – Procedures are for procedural tasks. There are too many tasks in our world to teach a procedure for each one. We do not have an infinite amount of memory. Do not teach procedures for conceptual tasks.
• Patsy Kanter and Steve Leinwand – The best way to teach the 9 multiplication facts is to do x 10 minus one group. (9 wants to be ten –nibbler 9) So 9 x 8 should be solved as 10 x 8 = 80 minus one group of 8. 80-8 = 72.
• Sherry Perish – Has written a Fraction, Decimal, and Percent number talk book, which should be out at the end of summer 2016. I can’t wait!
• Doug and Barbara Clarke – Researchers from Australia – Talked about productive struggle as controlled floundering or the zone of confusion. If genuine learning is to take place, you have to be in the zone of confusion.
• Max Ray – Have people give I notice / I wonder feedback. “I notice that you _______. That was awesome because _____________” “When you said _________________, I wondered __________________________.”
• Annie Fetter – Orally read a math task that is not posted for students. Have them share, what did you hear, what do you wonder to promote listening comprehension. She also talked about writing and the revision of writing in math. We revise our writing in ELA on improve our writing and improve the clarity of our thoughts. Why not write and revise in math?
• Lizzy Hull Barnes –Program Administrator for Mathematics and Richard Carranza, Superintendent – San Francisco Unified School District – In 2014 they passed a board policy and curriculum pathway that does not permit ability grouping until 11th grade. They have the highest math achievement on the Smarter Balanced assessment out of all large urban school districts in California. In every grade level in SFUSD, the number of students whom scored at or above grade level on the SBAC was above the state average in 2015. They believe that all students can learn and have data to support that heterogeneous groups in mathematics, do not take away from the achievement of the high students.
• Francis (Skip) Fennel and Gary Martin – Why not let students use calculators when there is 30 years of research that states that calculators do not take away from computational skills.
• Nicholas Gilbertson and Jia He – In-depth conceptual understanding of the division of fractions is not easy to develop.
• Loria Allen – Do a rich task everyday, and when we do, know what we are looking for from the students.
• Connie Schrock and Kit Norris – http://www.mathsisfun.com/games/broken-calculator.html is a really great game.
• Marilyn Burns – 1 on 1 interviews allow a glimpse into student thinking in a way you can not access with paper and pencil, especially with primary students.
• I also met with Sheela Sethuraman from CueThink. Great conversation about the use of technology and she made me wonder more about technology integration. I am looking forward to trying CueThink with students. My favorite implementation idea is having an older buddy class teach the program and give feedback on the work of little buddies.
• I wrapped up my conference with Ignite talks. Graham Fletcher was amazing. He very clearly stated, “If we want our students to talk more, we need to talk less.” Ask questions to help students develop understanding, and when they do share their thinking, stop rephrasing or clarifying their words. It takes away their ownership and tells the other students that they don’t have to listen to the ideas of their classmates.
What an amazing 3 days!
Quick Clips Common Core Math
Over the past few years I have heard over and over from parents that they just don’t understand how to help their children with their math homework anymore. I have heard similar concerns from teachers. How do parents help their children with strategies they have never seen or heard of before? Parent and producer, Les Mayfield approached me with his frustration and his proposed solution. From that conversation, Quick Clips common core math videos were born. We have created 36 strategy videos to help parents and teachers understand frequently used computational strategies.
